

This rule is commonly known as the antiderivative power rule.
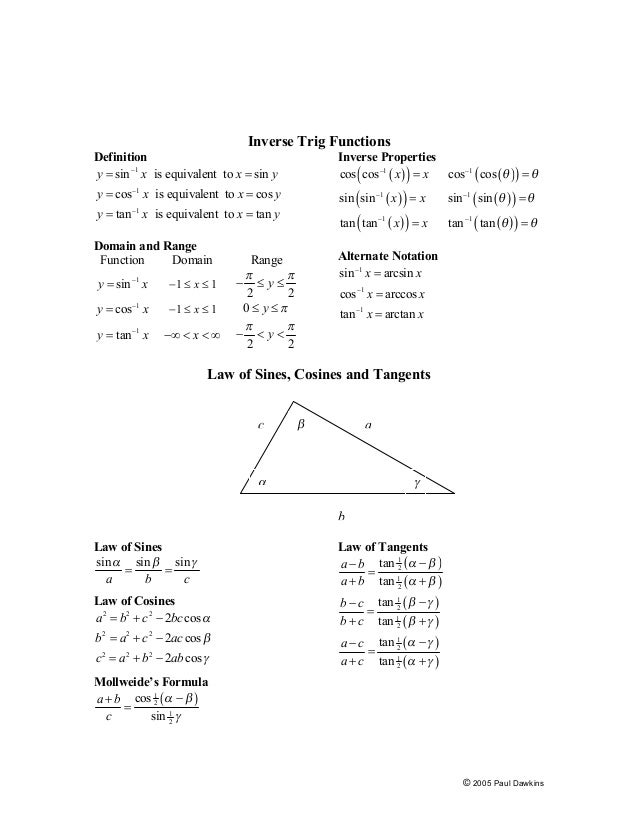
Now, the antiderivative rule of power of x is given by ∫x n dx = x n+1/(n + 1) + C, where n ≠ -1. The antiderivative rules are common for types of functions such as trigonometric, exponential, logarithmic, and algebraic functions. We will discuss the rules for the antidifferentiation of algebraic functions with power, and various combinations of functions. Recall also the isosceles right triangle and find the described ratios in itįor the rest of the first quarter just memorize that the common 30-60-90 degree triangle has its shortest to longest edge ratio of $1:2$ (see the image linked above) so sine reaches $1/2$ at one-third of the right angle.In this section, we will explore the formulas for the different antiderivative rules discussed above in detail. As it's symmetric it reduces to really only the three cases above.įirst of all, you know the square diagonal is $\sqrt 2$ of the side, so sine and cosine of $45^\circ$ is $1/\sqrt 2 = \sqrt 2/2$ and tangent is $1$. As the circular picture is perfectly symmetric it's easy to memorize even though it does, technically, have 48 values. Which values depend upon whether $|x| > |y|$ or $|y| > |x|$ and which quadrant $(x,y) lie in.Īnd yes, that circular picture helps. Unfortunately, you have to memorize the definitions of the sine and tangent: $\sin=\text$ The triangle has two sides of length $1$ if you have memorized the theorem of Pythagoras, you can figure out that the length of the third side is $\sqrt2.$ Either one of these triangles has angles of $90^\circ,45^\circ,45^\circ$ no need to memorize $45$, just divide $90$ by $2$. Next, cut the square along the diagonal, making two triangles. The square has four sides of equal length, which we take to be $1$. The trig functions for $30^\circ,45^\circ,60^\circ$ are based on two simple geometric figures: the square and the equilateral triangle. (Never memorized them myself.) I would expect a student to have enough understanding to be able to figure them out in seconds.

I would not expect a student to memorize trig functions of easy angles.


 0 kommentar(er)
0 kommentar(er)
